Solving the P – Q Experiment
Alan Barnard (at the 2006 TOC-ICO conference) asked the question whether the simple Throughput per Constraint Unit rule is valid with 2 (or more) overloaded resources. Alan used Eli Goldratt’s P-Q thought experiment for his discussion. His question is important because it is common to see businesses reduce ‘excess’ capacities to balance (or almost balance) capacities. The practice often results in two) or more concurrent constraints or ‘almost’ constraints. Since 2006 I have observed several factories that wonder why their output collapses below the theoretical capacity of their (almost) balanced lines.
I plan to show that that the Throughput per Constraint Unit rule continues to be valid using the same P-Q thought experiment. I also want to discuss this result in relation to the real World – how should companies manage resource capacities.
I would like readers to follow Eli Goldratt’s recommendation that they solve the problems – before I provide the solution and before my discussion of results. The learning experience will be greater and readers should be better able to discuss and critique my conclusions. If you are familiar with the thought experiment you can jump to the second part of this article.
The key part of the article comes at the end when the solution used in the P-Q thought experiment is discussed in relation to REALITY. The thought experiment should not lead managers to an easy solution. The tool is useful but requires thought and care.
Most of us ‘solve’ the problem without much thinking. It’s like a simple arithmetic problem from grade school. From groups we usually get quite number of ‘wrong’ answers – some from arithmetic mistakes, some from faulty thinking. Below you will find the answers from inadequate thinking and the explanation for the ‘right’ solution.
Attempt 1:
Many do not check whether or not my factory has sufficient capacity to produce all the Ps and Qs. They do not identify the constraint. These people, barring arithmetic errors come up with the answer shown below – 1500€ profit per week. That would be nice, but why would I ask a consultant for help? The constraint makes it impossible to earn 1500€.
So, let’s find the “Find the constraint!”
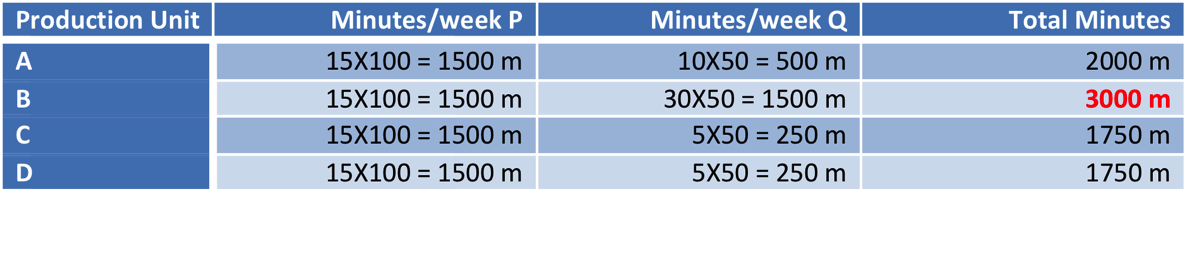
The table below identifies it.
Clearly the B machine cannot produce all of the necessary components for both P and Q. We need to decide how many Ps and how many Qs we can produce (the optimal mix) to maximise profit. B is the constraint and, in this case, also a bottleneck.
Attempt 2
Those that found the bottleneck usually ask things like ‘Can we buy another machine?’ or ‘Can we use overtime?’ For the purpose of the experiment no additional machine and no overtime is possible. The job is to maximise profit within the given parameters. To expand capacity by either overtime or adding a machine would mean we jump questions 2 and 3 of the 5 focusing steps.
Step 2 of the 5 steps is to decide how to exploit the constraint (our bottleneck). Most people, from high school students to CEOs, will check at least some of the following to see which product is the more profitable and should be favoured by the constraint:
- Which product has the higher price? Q (100 vs. 90€ for P)
- Which product has the higher contribution margin (Throughput[1])? Q (60 vs. 45€ for P)
- Which product requires the least amount of effort to produce it? Q (50 vs. 60 minutes of effort per unit of P)
Based on these 3 checks it looks like Q is the better choice. Most people choose Q as the more profitable product. We should, therefore, produce all the Q (50) and fill our bottleneck’s capacity with P sales.
If we sell 50Q we consume 1500 minutes of B capacity (2, 15 minute operations multiplied by 50 units of Q). We have 900 minutes of B capacity left – enough to produce 60 P units. The table below shows our result.
Not so good! Despite using commonly practiced checks for product profitability we generate a loss. Is this the best we can do? Might there a better way to decide what we should produce?
We did not apply the second of the 5 focusing steps correctly. The 3 checks we made had nothing to do with deciding how to exploit the bottleneck machine B. Maybe we should ask: How long does it take B to create 1 € of Throughput? How many minutes of B do each of our 2 products P & Q to require produce 1€ of Throughput?
- Q Throughput is 60€ per unit. It takes 30 minutes to produce this Throughput. Producing Q our resource B delivers 2 Euros of Throughput every minute. It takes 30 sec. of B’s time to produce 1€.
- P Throughput is 45€ per unit. It takes 15 minutes to produce these Euros. Producing P our resource B delivers 3 Euros every minute; it takes only 20 sec. to produce 1€ with P.
Shouldn’t our decision be to produce the product that delivers the greatest number of €s per unit of time available at B (the constraint; the limiting factor for Throughput)? (Alternatively shouldn’t the decision be to produce that product with which B delivers 1€ in the least amount of time?) If yes, then our decision must be to produce 100 P and 30 Q (100 P consume 1500min of B capacity, leaving 900min for Q. Since one Q requires 30min of B capacity only 30 can be produced). The table below shows the result.
It looks like we have found a nice rule to determine what we should sell when a bottleneck exists. Throughput per constraint unit will tell us which product to favour – bearing in mind that our customers and our markets may not allow us to reach the theoretical maximum.
During Alan Barnard's presentation in 2006 he raised the question whether this nice simple rule (deciding based on Throughput per Constraint Unit) always works. He introduced a second constraint (at D) and asked how much we can produce now that two constraints are active at the same time. Is our simple rule still valid? If not, can we adapt the rule so that (sales) managers continue to have something simple on which to base their preferred product mix? The next post will show the P Q thought experiment modified for a second constraint at D.

Chateau Frontenac, Québec City, May 1954,
[1] Throughput = the rate at which we make money = sales less totally variable cost (usually just materials).